The law of conservation of energy is a fundamental principle of physics that states that energy cannot be created or destroyed; it can only be transformed from one form to another. This law is crucial in understanding various natural and technological processes, ranging from mechanical movements to chemical reactions and nuclear transformations. This blog explores the law in detail, providing theoretical explanations, mathematical formulations, and real-world examples.
Historical Background
The concept of energy conservation has a long history, evolving through different stages of scientific discovery. The roots of this principle can be traced back to ancient Greece, where philosophers such as Aristotle speculated about the nature of motion and change. However, these early ideas lacked experimental validation and mathematical formulation. It was not until the 17th century that significant advancements were made. Galileo Galilei and Johannes Kepler contributed to the understanding of motion, paving the way for Isaac Newton’s laws of motion, which provided a mechanical framework for analyzing energy transformations. Newton’s work laid the foundation for classical mechanics, although the explicit formulation of energy conservation was still missing.
During the 18th and 19th centuries, further progress was made in understanding energy transformations. The work of Émilie du Châtelet was instrumental in refining the concept of energy, particularly by promoting the idea that energy is proportional to the square of velocity, an insight that later contributed to the development of kinetic energy equations. In the early 19th century, scientists such as Sadi Carnot, Julius Robert von Mayer, and James Prescott Joule established key principles of thermodynamics and energy conservation. Mayer proposed that heat and mechanical work were interchangeable, while Joule conducted experiments demonstrating the mechanical equivalent of heat. These contributions helped solidify the first law of thermodynamics, which formally states the conservation of energy principle.
By the late 19th and early 20th centuries, the conservation of energy principle was extended to include new discoveries in electromagnetism and quantum mechanics. Hermann von Helmholtz mathematically formulated the conservation law, integrating it into broader physical theories. The development of Albert Einstein’s theory of relativity in the early 20th century introduced the famous equation , which showed that mass itself is a form of energy, leading to the realization that energy conservation applies even in nuclear reactions. This groundbreaking discovery revolutionized physics and had profound implications for modern science, including nuclear energy and particle physics. The historical evolution of energy conservation has thus shaped our understanding of the universe and remains a cornerstone of scientific exploration.
Mathematical Formulation
The law of conservation of energy can be mathematically represented as:
E total = E kinetic + E potential + E others
where:
- E kinetic is the kinetic energy,
- E potential is the potential energy, (gravitational potential energy)
- E others represents other forms of energy such as thermal, chemical, or nuclear energy.
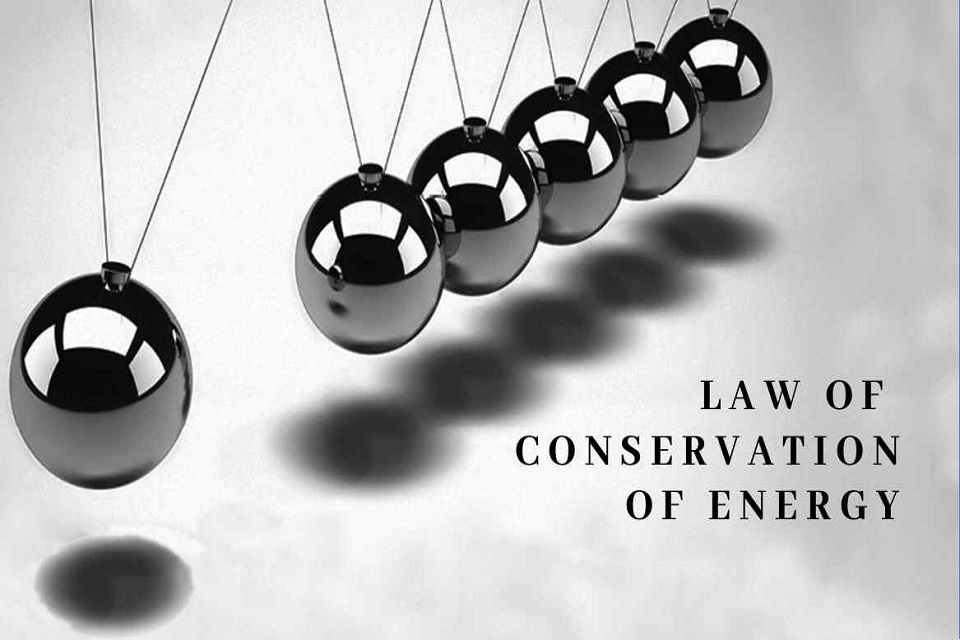
For a closed system with no external work done, the total energy remains constant: This principle applies universally, governing everything from planetary motion to biochemical processes in living organisms.
Types of Energy and Their Conservation
Mechanical Energy
- When an object falls, gravitational potential energy is converted into kinetic energy.
- Example: A roller coaster moving from the highest point to the lowest.
Thermal Energy
- Energy transfer occurs due to temperature differences, but the total energy remains conserved.
- Example: Friction converts mechanical energy into heat energy.
Chemical Energy
- Stored in molecular bonds and released through chemical reactions.
- Example: Combustion of fuel converts chemical energy into heat and kinetic energy.
Electrical Energy
- Conversion between electrical and mechanical energy in generators and motors.
- Example: A battery powering an electric circuit transforms chemical energy into electrical energy.
Nuclear Energy
- Mass-energy equivalence (E = mc2) shows how nuclear reactions conserve energy by converting mass into energy.
- Example: Nuclear fission in power plants or fusion in the sun.
The law of conservation of energy is a cornerstone of physics, with extensive implications across disciplines. Understanding this principle allows for advancements in technology, sustainability, and scientific exploration. Whether in natural phenomena or engineered systems, energy transformations dictate the behavior of our universe, reaffirming the immutable nature of this fundamental law.